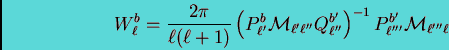Back to Main Archeops Page Back to Main Journal Page

Archeops C(l) data :
These tables correspond to the RED points in the C(l) paper.

C(l)
covariance matrix
window functions

Power Spectrum for Archeops experiment KS3 results (astro-ph/0210305) as used in astro-ph/0210306

You can find in this directory two files containing the power spectrum for the Archeops power spectrum (in microK^2) for each of our 16 bins.

Table contains 6 columns and 16 rows : ellmin, ellmax, spectrum, err_spectrum, nu, beta^2
Units are microK^2

Nu and beta are approximated in the following way (Bartlett et al. 2000 A&AS 146 506)
Likelihood = X^nu/2 exp(-X/2)
with X = nu * (spectrum+beta^2)/(Cl+beta^2)

Jean-Christophe Hamilton, Marian Douspis - oct. 22, 2002
email : hamilton@in2p3.fr and douspis@astro.ox.ac.uk

FITS table

ASCII table

Covariance Matrix for Archeops experiment KS3 results (astro-ph/0210305)

You can find in this directory two files containing the covariance matrix for the Archeops power spectrum (in microK^4). It is a 16x16 matrix (16 bins). The correlation of the last bin with the others is set to zero as this bin is computed with a different weighting of the maps (see article, section 3.3)

Table contains 16x16 array

Units are microK^4. the error bars for each bin can be computed from the square root of the diagonal of the covariance matrix.

Jean-Christophe Hamilton - oct. 22, 2002
email : hamilton@in2p3.fr

FITS table

ASCII table

Window Functions for Archeops experiment KS3 results (astro-ph/0210305)

You can find in this directory two files containing the window functions for the Archeops power spectrum.

The integral of the window functions are normalized to 1 for each of our 16 bins and are computed between l=0 and l=768.

Table contains float array with dimension 16*769 (16 columns of 769 rows)

The definition we use here for the window functions is the following (with implicit sum over repeated indices):

$\begin{displaymath}\mathcal{C}_b=W_\ell^b \left[ \frac{\ell(\ell+1)}{2\pi}C_\ell \right]\end{displaymath}$

(1)

with the notation :

$\begin{displaymath}\mathcal{C}_\ell=\frac{\ell(\ell+1)}{2\pi}C_\ell\end{displaymath}$

(2)

In the MASTER framework (Hivon et al., 2002), if we define $\mathcal{M}$ as :

$\begin{displaymath}\mathcal{M}_{\ell^\prime \ell^{\prime\prime}}=M_{\ell^\prime......\prime\prime}}F_{\ell^{\prime\prime}}B_{\ell^{\prime\prime}}^2\end{displaymath}$

(3)

then the window function reads:

$\begin{displaymath}W_\ell^b=\frac{2\pi}{\ell(\ell+1)}\left(P^b_{\ell^\prime}\ma......rime\prime\prime}}\mathcal{M}_{\ell^{\prime\prime\prime} \ell}\end{displaymath}$

(4)

where

and

are the the binning and reciprocal operators.

Using this definition, we have for each bin :

$\begin{displaymath}\sum_\ell W_\ell^b=1\end{displaymath}$

(5)

Jean-Christophe Hamilton & Simon Prunet- nov 05, 2002
email : hamilton@in2p3.fr prunet@iap.fr